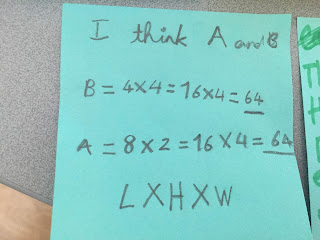Here is the invitation to the prompt:
And here is what the volumes looked like.
The first had 250mL, the second had 500mL, the third had 750mL and the fourth 1 . 5 litres.
It was really interesting to observe and listen into the reasoning discussions they were having amongst each other.
Some children drew upon prior knowledge about how much water adults need to consume and so they adjusted that amount to what they thought a child might require.
Some tried to estimate how much they personally would drink in a day and tried to match that estimate with a volume.
A few children began discussing whether they had to actually drink that volume or could the water also be included in the food they eat.
Some others used reasoning skills to try to determine whether it would be 750 mL or 1. 5 litres. They had already eliminated the two smaller volumes as they felt they drank that much before lunch time. Their gut instinct was telling them that the 1 . 5 litres looked like A LOT of water, so perhaps it was more reasonable to think the 750 mL would be the amount.
One student had remembered reading that adults need to drink 8 cups per day, so he found a cup at our sink and brought it over beside the litre beaker to estimate how many cups that would equate to. He later decided to return to the sink and poured 8 cups of water into another litre jug to test his hypothesis.
Some of our thoughts:
I liked this strategy of comparing his water bottle to the volumes of water shown:
Sharing prior knowledge about water being found in food:
Testing his own hypothesis based on prior knowledge:
There was a lot of genuine engagement and sharing of theories with this prompt.
Later in the day, we discussed what we already knew about why we need to constantly drink water throughout the day.
In our class, we have a volunteer who is our 'Rehydrator of the Week'. Throughout the day, this person will raise their water bottle and shout out 'Cheers!' to the class. When we hear that, we all raise our water bottles and say 'Cheers!' and then thank the rehydrator for reminding us to have a drink.
This is a fun routine we have, but it also (hopefully) is helping to create a habit for the children to be conscious of drinking water regularly.
Over the year, we have discussed often the impacts of drinking water often to help our brains think to their best ability etc.
We also have a class 'energiser'. This person's responsibility is if they feel we have been sitting down for too long, they invite us to the front of the room and will demonstrate three body movements which we copy. The purpose of this is to help circulate the blood in our bodies which e understand carries oxygen to our brains that helps us to think more effectively. I'm hoping this routine will help create positive habits of staying alert and healthy.
We then watched this TedTalk about the benefits of drinking water and discussed some of the information it shared and our wonderings that emerged from it.
We then looked at our water volume prompt and shared some of the strategies we used to determine how much water we should drink. Some of us were pretty shocked to see it was approximately 1 . 5 litres because it does, indeed look a lot!
In our discussion, some children shared some personal action plans to try to ensure they do drink more water throughout the day which was great to hear (even without our class rehydrator reminding us)
Another suggested we find a way to measure how much water we consume per day to see if we ae being healthy or not and so next week, we will use that idea and try to create a way to record this. This is a great example of giving children opportunities to take action with their learning. It will be interesting to see how our class decides to go about this.
I think these morning prompt routines are a really effective way to engage children in mathematical thinking. They are not mandatory to participate in which I think makes them work better. The children who choose to participate are genuinely engaged and that engagement (I suspect) creates more interest in those other children who might normally pass on the maths thinking opportunity.